Cubics

Thursday 26 January 2006 at 9:26 pm | In Articles | 1 Comment

A problem that I give some of the students is to show that for a cubic f that if x=\alpha and x=\beta are the x-coordinates of its stationary points then f^{\prime\prime}( \alpha ) =-f^{\prime \prime}( \beta ). Hopefully, they will have already noticed this result in the exercises they have done.

I then ask them to show that the point of inflexion of f is at the midpoint of the turning points. This involves using the equations for the roots of a quadratic: \alpha + \beta=-\frac{b}{a} and \alpha \beta=\frac{c}{a} but the algebra isn’t particularly nice (details are here).

It dawned on me that there’s a better way which is not only simpler to prove but gives a more powerful result: a cubic is (rotationally) symmetric about its point of inflexion.

To see this, take the cubic and translate it so that the point of inflexion is at the origin. This clearly has no effect on the symmetry so it is sufficient to prove the result in this case.

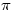If f(x)=ax^3+bx^2+cx+d has its point of inflexion at the origin then clearly d=0 and, as f^{\prime\prime}(0)=2b, it follows that b=0 so f(x)=ax^3+cx. Rotate this by \pi about the origin, for example by applying the usual matrix \begin{pmatrix} -1 & 0 \\ 0 & -1 \end{pmatrix}, and you get -f(x)=-ax^3-cx which is clearly the same cubic. Hence it has rotational symmetry about the origin, and in particular, the point of inflexion at the origin is the midpoint of the stationary points.

1 Comment »

RSS feed for comments on this post. TrackBack URI

  1. Nice result!

    Here’s an interesting problem based on the graph of a cubic.

    Take the graph of y = x^3. Pick point A anywhere on the curve other than the point of inflexion (the origin.) Draw the tangent to the curve at A, and continue until you meet the curve again at B. Now draw the tangent to the curve at B, and continue until you meet the curve at C.

    Show that, no matter the coordinates of A, the area between the curve and the line joining B and C is a fixed multiple (to be found) of the area between the curve and the line joining A and B.

    Comment by Nick — Tuesday 31 January 2006 3:16 am #

Leave a comment

XHTML: <a href="" title=""> <abbr title=""> <acronym title=""> <b> <blockquote cite=""> <cite> <code> <del datetime=""> <em> <i> <q cite=""> <s> <strike> <strong>

Powered by WordPress with Pool theme design by Borja Fernandez.
Entries and comments feeds. Valid XHTML and CSS. ^Top^