Mathematics Weblog
Blackboards
Tuesday 14 March 2006 at 12:58 pm | In Articles | 1 CommentThanks to Gooseania (who got it from Blog of a Math Teacher) for linking to The Sarong Theorem Archive. Just shows that blackboards still exist; they have completely vanished from FE and schools in the UK to be replaced by whiteboards or smartboards.
The problem is that whiteboards need regular proper cleaning and decent pens; smartboards are useless for maths since they are usually far too small and are too dependent on technology. I don’t miss the chalk dust but there’s little to beat a decent blackboard for flexibility.
Proof
Wednesday 15 February 2006 at 6:58 pm | In Articles | 3 CommentsFor a mathematician’s (Marcus du Sautoy) view of the recently released film Proof have a look at Another view. The film was not given a good review by the critics that I have read.
The Art of Justin Mullins – Beautiful Equations
Wednesday 1 February 2006 at 12:46 pm | In Articles | 1 CommentJustin Mullins frames beautiful equations – you can see them on his site www.justinmullins.com and in his exhibition in Highgate in London from now until 12th February. No prizes for guessing which equation takes pride of place!
The Guardian reports this exhibition at Mathematician defines beauty in new exhibition.
Goldbach and Lewis
Tuesday 31 January 2006 at 9:41 pm | In Articles | 1 CommentIt’s not often that mathematics makes an appearance on British TV so I was suprised to see it in Lewis, ITV’s follow up to the Inspector Morse series (see for example Lewis, and the Ghost of Inspector Morse and Newsnight Review).
Oxford University often appeared in the Morse programmes and this time in Lewis it featured a very bright student who had shown that a Fields’ medallist work on the Goldbach Conjecture was flawed, leading to all sorts of murders. Along the way we were shown what a perfect number was, as perfect numbers were used for passwords.
It’s a pity that the mathematics shown on one of the whiteboards was elementary coordinate geometry :). The programme showed how much passion mathematics can arouse, though I wouldn’t have thought it has ever lead to murder!
Mathematics Today Article – Maths Blogs
Monday 30 January 2006 at 12:52 pm | In Articles | Post CommentCraig Laughton, aka Gooseania, has written an article about mathematics blogs in Mathematics Today. Do go to his blog Exploring the blogosphere and read the article and provide the feedback and discussion he asks for.
Cubics
Thursday 26 January 2006 at 9:26 pm | In Articles | 1 CommentA problem that I give some of the students is to show that for a cubic  that if
that if  and
and  are the x-coordinates of its stationary points then
are the x-coordinates of its stationary points then  . Hopefully, they will have already noticed this result in the exercises they have done.
. Hopefully, they will have already noticed this result in the exercises they have done.
I then ask them to show that the point of inflexion of  is at the midpoint of the turning points. This involves using the equations for the roots of a quadratic:
is at the midpoint of the turning points. This involves using the equations for the roots of a quadratic:  and
and  but the algebra isn’t particularly nice (details are here).
but the algebra isn’t particularly nice (details are here).
It dawned on me that there’s a better way which is not only simpler to prove but gives a more powerful result: a cubic is (rotationally) symmetric about its point of inflexion.
To see this, take the cubic and translate it so that the point of inflexion is at the origin. This clearly has no effect on the symmetry so it is sufficient to prove the result in this case.
If  has its point of inflexion at the origin then clearly
has its point of inflexion at the origin then clearly  and, as
and, as  , it follows that
, it follows that  so
so  . Rotate this by
. Rotate this by 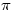 about the origin, for example by applying the usual matrix
about the origin, for example by applying the usual matrix  , and you get
, and you get  which is clearly the same cubic. Hence it has rotational symmetry about the origin, and in particular, the point of inflexion at the origin is the midpoint of the stationary points.
which is clearly the same cubic. Hence it has rotational symmetry about the origin, and in particular, the point of inflexion at the origin is the midpoint of the stationary points.
Latest Prime Found
Wednesday 28 December 2005 at 2:17 pm | In Articles | 3 CommentsUsing 700 computers a new Mersenne prime has been found. It is  and has 9,152,052 digits. The $100,000 prize for a prime with more than 10 million digits is still up for grabs!
and has 9,152,052 digits. The $100,000 prize for a prime with more than 10 million digits is still up for grabs!
More at ZDNet News and GIMPS.
Problem
Saturday 10 December 2005 at 8:32 pm | In Articles | 4 CommentsThere was a nice little problem posted on S.O.S. Mathematics CyberBoard which comes from an International Mathematics Tournament of Towns question:
- The least common multiple of positive integers
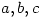 and
and  is equal to
is equal to 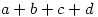 . Prove that the product
. Prove that the product  is divisible by at least one of
is divisible by at least one of 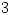 and
and 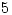 .
.If you need a hint you’ll find one on the AoPS Math Forum
Singapore
Thursday 8 December 2005 at 10:25 pm | In Articles | Post Commenteon gives a brief but fascinating look into Science and Maths in Singapore. Perhaps eon can be encouraged to expand on this.
Gratuitous Mathematics
Thursday 1 December 2005 at 7:11 pm | In Articles | 4 Comments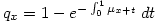
The meaning of life? (Not quite but this formula will tell you much of it you have left*)
That’s how the Guardian today publicised its article on life expectancy. It then attempted to ‘explain’ the formula with a tiny note saying *Details overleaf. These ‘details’ were:
- The force of mortality at age
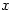 is defined as:
is defined as: 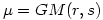 , with parameters
, with parameters  and
and  fitted by maximum likelihood. For example,
fitted by maximum likelihood. For example, 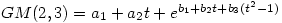 where
where  . The the equation above shows the probability that someone aged
. The the equation above shows the probability that someone aged 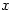 will die within one year. Still puzzled? See page 8.
will die within one year. Still puzzled? See page 8.On page 8 is the article So, how long have we got? which sheds no light on the formula; it doesn’t even mention it.
So why was this formula there? To make the journalist or the paper look clever? Who knows. But it certainly is completely useless even to mathematicians. The example just makes the whole thing even more obscure. Wouldn’t it be nice to have more mathematically trained journalists who wouldn’t just show off but instead make things clearer?
Powered by WordPress with Pool theme design by Borja Fernandez.
Entries and comments feeds.
Valid XHTML and CSS. ^Top^