Mathematics Weblog
This site uses LatexRender for the mathematics
Divisors
Sunday 30 January 2005 at 8:25 pm | In Articles | 2 CommentsMost people, when asked how many divisors the number 60 has (including 1 and 60), would struggle to do so without listing them all. Yet once you know that the prime factorisation of 60 is  you can immediately say that the number of divisors is
you can immediately say that the number of divisors is  . In other words, you take each index, add 1 then multiply them together.
. In other words, you take each index, add 1 then multiply them together.
This is easy to see if you list the divisors as

It’s then not difficult to prove the general result:
- If
 then the number of divisors of
then the number of divisors of 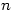 is
is 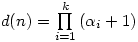
2 Comments »
RSS feed for comments on this post. TrackBack URI
Leave a comment
Powered by WordPress with Pool theme design by Borja Fernandez.
Entries and comments feeds.
Valid XHTML and CSS. ^Top^
I really like this result.
You can use it to show that the numbers with an odd number of factors must be square.
Comment by Ronald — Sunday 30 January 2005 10:13 pm #
I saw something like this while preparing for a math contest. It’s an interesting little result–I used something very similar to it to find the number of perfect square divisors of a given number.
Comment by Anna — Wednesday 2 February 2005 10:19 pm #