Mathematics Weblog

LaTeX

Sunday 30 May 2004 at 1:18 pm | In Articles | Post Comment

$\LaTeX$ is a very powerful language and allows one to type very nice mathematics in a way that would be difficult to do any other way. It is what provides the mathematics on this site. How else could one show

$\zeta(n)=\underbrace{\int_0^1 \cdots \int_0^1}_{n}\dfrac{\prod_{i=1}^n dx_i}{1-\prod_{i=1}^n x_i}$

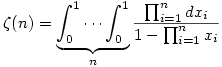
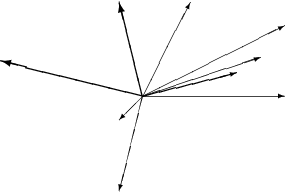
But $\LaTeX$ will do more than that and also provide graphics. See the diagram in Monday 10 May or how about these:

$\setlength{\unitlength}{0.5cm} \begin{picture}(5,2) \put(5,0){\line(-1,0){5}} \put(0,0){\line(1,1){2}} \put(2,2){\line(3,-2){3}} \put(-0.5,-0.1){\small A} \put(1.9,2.1){\small B} \put(5.1,-0.1){\small C} \put(4,0.1){\tiny 30} \qbezier(3.8,0)(3.9,0.55)(4.1,0.55) \put(0.6,1){\footnotesize 2} \put(3.6,1){\footnotesize 3} \put(2.3,-0.6){\footnotesize 5} \put(6,-0.2){\tiny \shortstack{t\r\i\a\n\g\l\e}} \end{picture}$

go on – spot the deliberate error 😕

$\setlength{\unitlength}{1mm} \begin{picture}(60,40) \put(30,20){\vector(1,0){30}} \put(30,20){\vector(4,1){20}} \put(30,20){\vector(3,1){25}} \put(30,20){\vector(2,1){30}} \put(30,20){\vector(1,2){10}} \thicklines \put(30,20){\vector(-4,1){30}} \put(30,20){\vector(-1,4){5}} \thinlines \put(30,20){\vector(-1,-1){5}} \put(30,20){\vector(-1,-4){5}} \end{picture}$

See here for a good tutorial on $\LaTeX$ graphics

Two Interesting Functions

Sunday 23 May 2004 at 10:33 am | In Articles | 2 Comments

At A level students only see reasonably nice functions, though occasionally they come across

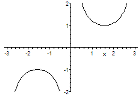
where the maximum is smaller than the minimum (which, incidentally, is why one should use the terms local maximum and local minimum instead).

But what they won’t have come across are functions like these:

$f(x)=\left\{\begin{array}{ll}1 & \mbox{ if } x \mbox{ is rational}\\0 & \mbox{ if } x \mbox{ is irrational}\end{array}\right.$

$g(x)=\left\{\begin{array}{ll}1/q & \mbox{ if } x = p/q,\ q>0, \mbox{ in lowest terms}\\0 & \mbox{ if } x \mbox{ is irrational}\end{array}\right.$

When trying to draw the graphs remember that

between every pair of irrational numbers there is a rational number

between every pair of rational numbers there is a irrational number

You can very roughly draw f using dots and there’s a nice picture of g at mathworld
Now think about integrating f and g – do they have areas under between them and the x-axis? The answer to ths question is important in the theory of integration.

These functions have other interesting properties and illustrate the relationship between rational and irrational numbers.

Nice Problem

Sunday 16 May 2004 at 7:28 pm | In Articles | Post Comment

There’s a very nice problem on The University of Warwick Mathematical Society’s site.
I won’t say exactly where because it has the solution there as well 😕

If is continuously differentiable on the open interval
$\lim_{x \to a^{+}}f(x)=\infty$

$\lim_{x \to b^{-}}f(x)=-\infty$

$f^{\prime}(x)+f(x)^2 \geq 1$

then prove that $b-a \geq \pi$

Stuck!. Then click on read more for a hint
Continue reading Nice Problem…

Going dotty

Monday 10 May 2004 at 6:59 pm | In Articles | Post Comment

A well-known problem is to be given 9 dots

$\setlength{\unitlength}{0.5cm} \begin{picture}(7,7) \multiput(0,0)(1,0){3}{\circle*{0.15}} \multiput(0,1)(1,0){3}{\circle*{0.15}} \multiput(0,2)(1,0){3}{\circle*{0.15}} \thinlines \end{picture}$

You have to connect the dots by using 4 lines, without taking your pen off the paper and only going through each dot once. At first sight this looks impossible until you are shown you can go outside the square formed by the dots as in

$\setlength{\unitlength}{0.5cm} \begin{picture}(7,7) \multiput(0,0)(1,0){3}{\circle*{0.15}} \multiput(0,1)(1,0){3}{\circle*{0.15}} \multiput(0,2)(1,0){3}{\circle*{0.15}} \thinlines \put(1,2){\line(-2,0){2}} \put(-1,2){\line(1,-1){3}} \put(2,-1){\line(0,1){3}} \put(2,2){\line(-1,-1){2}} \end{picture}$

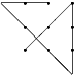
But what about 16 dots arranged in a square, or 12 dots arranged in 3 rows of 4? How many lines are needed for a 10 x 10 grid? What if arcs of circles are used instead of lines?

These and other fascinating questions about dots can be found at the web page simply called dots

Problem

Monday 26 April 2004 at 4:53 pm | In Articles | 4 Comments

Firstly, show that:

$\log_{a^n}b^n=\log_{a}b$

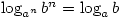
Secondly, are there any other operations you can do to 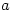and 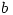and the $\log$ retains the same value? In other words:

Find all functions

$f,g:\mathbb{R^+}\mapsto \mathbb{R^+}$

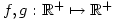such that

$\log_{f(a)}g(b)=\log_a b$

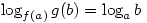for all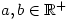

$a,b\in\mathbb{R^+}$

Cancelling

Tuesday 20 April 2004 at 4:27 pm | In Articles | 1 Comment

Students love to cancel wherever they can, so much so that the book Comic Sections (now out of print) had the following joke:

The student law of universal cancellation: If the same symbol x occurs in any two different places on the one page it may be cancelled

So you get horrors like $\dfrac{\sin x}{n}=\dfrac{\text{si}\hspace{-1mm}\not{n}\hspace{1mm} x}{\not n}=$ six$$ 😮

And yet strange things can happen. It is true that $\frac{2666}{6665}=\frac{266}{665}=\frac{2}{5}$ .
What is remarkable is that you can have as many sixes as you like and cancel them as many times as you like so, for example, $\frac{26666666}{66666665}=\frac{266}{665}$ . Can you prove this is true? Can you find 3 other similar fractions?

Think about it!

Sunday 18 April 2004 at 5:07 pm | In Articles | 2 Comments

What is the value of $(x-a)(x-b)(x-c)\dots(x-z)$ ?

The LambertW Function

Friday 9 April 2004 at 2:35 pm | In Articles | 1 Comment

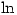Many equations cannot be solved exactly without using special functions. For example, to solve 2^x=3 requires the use of the $\ln$ function (or similar). This function is sometimes defined in terms of an integral from which their properties can be deduced. Thus 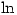$\ln$ is defined by $\displaystyle \ln x=\int ^x _1 \dfrac{dt}{t}\text{ for } x>0$ and it is then clear that, for example, $\ln 1=0$

There are many equations that can only be solved in terms of newly-defined functions. One such function that isn’t all that well known is the LambertW function where $w\left(x\right)$ is defined as a solution (for ) of we^w=x . This allows you to solve equations like 2^x=x^8 which was asked about on the S.O.S. Mathematics CyberBoard

To solve 2^x=x^8 let $w=-\ln x$ so that $x=e^{-w}$ . Then

$2^x=x^8 \Longrightarrow 2^{e^{-w}}=e^{-8w} \Longrightarrow e^{-w}\ln 2=-8w \Longrightarrow -\frac{1}{8}\ln 2=we^w$

Thus $w=w(\frac{1}{8}\ln 2)$ and so $x=e^{-w}=-8\dfrac{w(\frac{1}{8}\ln 2)}{\ln 2}$ which is our answer.

Using tables or software this gives 1.100.

But hang on, is that the only solution? No, because 2^x<x ^8 for small values of 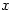and 2^x grows much faster than x^8 so 2^x>x^8 for large values of 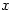. Since both $x \mapsto 2^x$ and $x \mapsto x^8$ are continuous on $\mathbb{R}$ there is another value of 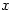for which 2^x=x^8 . A quick fiddle with a calculator gives $x\approx43.5$ .

Research into the LambertW function to find out how this other solution can be given in terms of this function.

Joke

Sunday 4 April 2004 at 2:25 pm | In Articles | Post Comment

Joi Ito’s Page contains a wonderful joke which I really have to repeat here.

$\displaystyle \lim_{x \to 8} \dfrac{1}{x-8}=\infty$

$\displaystyle \lim_{x \to 5} \dfrac{1}{x-5}= \rotatebox{90}{5}$

😀

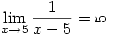
Trig Ratios

Sunday 4 April 2004 at 10:55 am | In Articles | 1 Comment

A level syllabuses these days expect you to remember the exact values of sin cos and tan of certain angles. 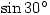$\sin 30^\circ$ is easy enough as the calculator will give you the exact answer, but unless you know roughly what 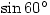$\sin 60^\circ$ should be then the calculator will be no help.

But, help is at hand 😀 Memorising formulae is easier when there’s a pattern and the following table gives such a pattern.

$\begin{array}{|c|c|c|c|c|c|} \hline \theta & 0^\circ & 30^\circ & 45^{\circ} & 60^\circ & 90^\circ \\ \hline \begin{array}{c} \\ \sin \theta \\ \\ \end{array} & \dfrac{\sqrt{0}}{2} & \dfrac{\sqrt{1}}{2} & \dfrac{\sqrt{2}}{2} & \dfrac{\sqrt{3}}{2} & \dfrac{\sqrt{4}}{2} \\ \hline \begin{array}{c} \\ \cos \theta \\ \\ \end{array} & \dfrac{\sqrt{4}}{2} & \dfrac{\sqrt{3}}{2} & \dfrac{\sqrt{2}}{2} & \dfrac{\sqrt{1}}{2} & \dfrac{\sqrt{0}}{2} \\ \hline \end{array}$

Isn’t that amazing! I only came across this a few years ago but apparently it’s been around at least since the 1950’s.

What about tan? Since 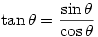$\tan \theta = \dfrac{\sin \theta}{\cos \theta}$ you just divide a value from the second row by the one below it (but please not for 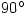$90^\circ$ ; see Tuesday 16 March).

« Previous Page — Next Page »